Display Title
Definition--Sequences and Series Concepts--Arithmetic Sequence
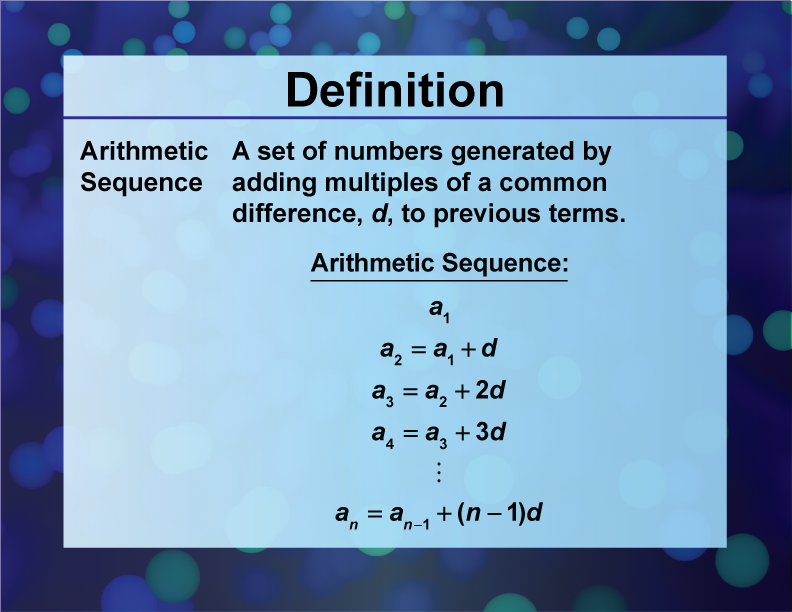
Display Title
Definition | Sequences and Series Concepts | Arithmetic Sequence
This is part of a collection of definitions related to sequences, series, and related topics. This includes general definitions for sequences and series, as well as definitions of specific types of sequences and series, as well as their properties.
—CLICK PREVIEW TO SEE THE DEFINITION—
To see the complete set of definitions on this topic, click on this link.
The following section includes background information on sequences. Use it to provide additional context for the set of definitions.
Sequences
Some number patterns are examples of sequences. A sequence is a set of numbers generated by applying the same rule to each term in the sequence.
Here is an example of a sequence.
In the example above, the rule “add 2” is applied to each term to generate the next term.
Arithmetic Sequences
An arithmetic sequence involves adding or subtracting the same amount to each subsequent term.
Here’s an example of an arithmetic sequence that involves subtraction.
With an arithmetic sequence, the term that keeps being added or subtracted is called the common difference. Here is an example of an arithmetic sequence with the common difference indicated.
The terms of an in a sequence can be listed symbolically, as shown below.
The first term is a1, the second term is a2, and so on to an. Any arithmetic sequence can be written this way.
Why would we write sequences this way? It makes it easier to see how each subsequent term is built from the previous term, as shown below.
The general form of an arithmetic sequence is known as a recursive formula. Furthermore, you can see how each term in the sequence is really based on two values, a1 and the common difference, c. This results in a different formula, shown below.
This form of the sequence equation is known as an explicit formula, as shown below.
Explicit formulas are extremely useful for finding any term in the sequence, as shown below.
Geometric Sequences
Another type of sequence is called a geometric sequence. Instead of adding or subtracting a number to generate terms, use multiplication. Here is an example.
This is the recursive formula for finding the nth term of a geometric sequence.
This is the explicit formula for a geometric sequence.
Note: The download is a PNG file.
Related Resources
To see additional resources on this topic, click on the Related Resources tab.
Create a Slide Show
Subscribers can use Slide Show Creator to create a slide show from the complete collection of math definitions on this topic. To see the complete collection of definitions, click on this link.
To learn more about Slide Show Creator, click on this Link.
Accessibility
This resources can also be used with a screen reader. Follow these steps.
-
Click on the Accessibility icon on the upper-right part of the screen.

-
From the menu, click on the Screen Reader button. Then close the Accessibility menu.

-
Click on the PREVIEW button on the left and then click on the definition card. The Screen Reader will read the definition.
| Common Core Standards | CCSS.MATH.CONTENT.6.SP.B.4, CCSS.MATH.CONTENT.HSF.IF.A.3, CCSS.MATH.CONTENT.HSF.BF.A.2, CCSS.Math.CONTENT.HSF.LE.A.2 |
|---|---|
| Grade Range | 6 - 9 |
| Curriculum Nodes |
Algebra • Sequences and Series • Sequences |
| Copyright Year | 2013 |
| Keywords | data analysis, arithmetic sequence, common difference, definitions, glossary terms, geometric sequence, common ratio |