Display Title
Definition--Calculus Topics--Absolute Value Function
Display Title
Definition--Calculus Topics--Absolute Value Function
Definition--Calculus Topics--Absolute Value Function
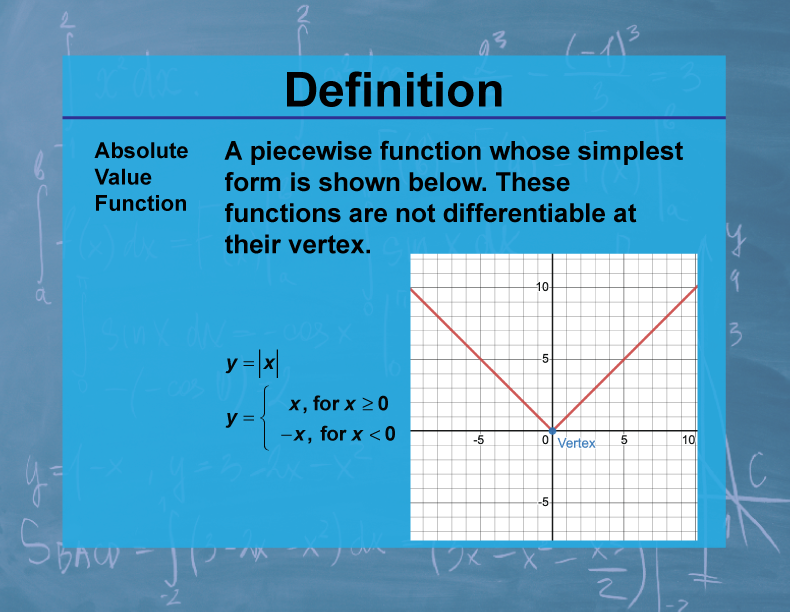
A piecewise function whose simplest form is shown below. These functions are not differentiable at their vertex.
— CLICK THE PREVIEW BUTTON TO SEE THE DEFINITION CARD —
To see the complete collection of Calculus terms, click on this link.
| Common Core Standards | CCSS.MATH.CONTENT.HSF.IF.C.7, CCSS.MATH.CONTENT.HSF.BF.A.1.C |
|---|---|
| Grade Range | 11 - 12 |
| Curriculum Nodes |
Algebra • Advanced Topics in Algebra • Calculus Vocabulary |
| Copyright Year | 2023 |
| Keywords | calculus concepts, limits, derivatives, integrals, composite functions |