Display Title
Definition | 3D Geometry Concepts | Horizontal Cross-Sections of a Square Pyramid
Display Title
Definition | 3D Geometry Concepts | Horizontal Cross-Sections of a Square Pyramid
Definition | 3D Geometry Concepts | Horizontal Cross-Sections of a Square Pyramid
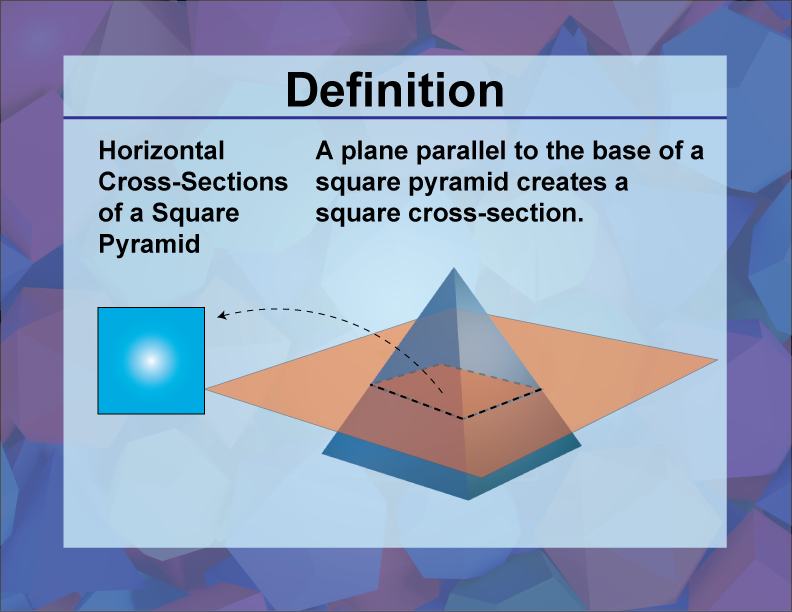
This is a collection of definitions related to 3D figures and their properties. This includes definitions and examples of 2D nets of 3D figures, as well as 2D cross-sections of different 3D figures.
Note: The download is a PNG file.
Related Resources
To see additional resources on this topic, click on the Related Resources tab.
Create a Slide Show
Subscribers can use Slide Show Creator to create a slide show from the complete collection of math definitions on this topic. To see the complete collection of definitions, click on this Link.
To learn more about Slide Show Creator, click on this Link.
Accessibility
This resources can also be used with a screen reader. Follow these steps.
-
Click on the Accessibility icon on the upper-right part of the screen.

-
From the menu, click on the Screen Reader button. Then close the Accessibility menu.

-
Click on the PREVIEW button on the left and then click on the definition card. The Screen Reader will read the definition.
| Common Core Standards | CCSS.MATH.CONTENT.5.MD.C.3, CCSS.MATH.CONTENT.7.G.A.3 |
|---|---|
| Grade Range | 4 - 6 |
| Curriculum Nodes |
Geometry • 3D Geometry • Pyramids |
| Copyright Year | 2021 |
| Keywords | three-dimensional geometry, 3d Geometry, defnitions, glossary term |