Linear Functions and Hooke's Law
A simple application of linear functions is Hooke’s Law, which involves a physics application. The reason Hooke’s Law is a simpler application to start with is because it is a direct variation of the form
f(x) = kx
Graphs of these types of functions cross the origin and in teaching about the slope-intercept form, it’s always easier to start with a y-intercept of zero.
So, the math is manageable, but so is the physics! The best example of Hooke’s Law involves the compression or stretching of springs. You don’t need a complicated lab set up to show the properties of springs. In fact, you don’t even need a prop, as an illustration will work fine.
You can use this animated GIF to show the basic idea. Start with a spring, unstretched. Then attach a unit of weight. Notice that the spring stretches a certain length, x.
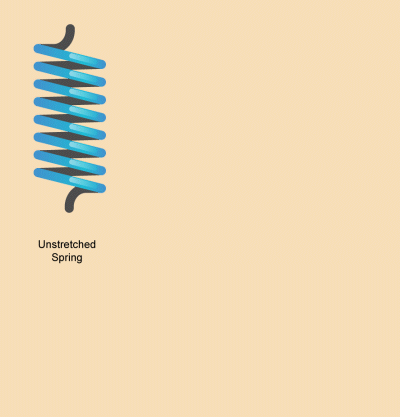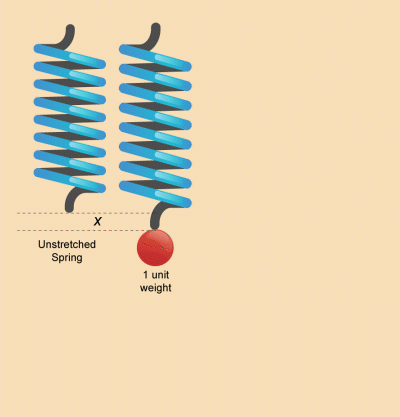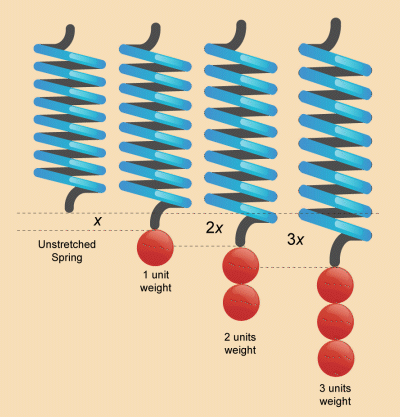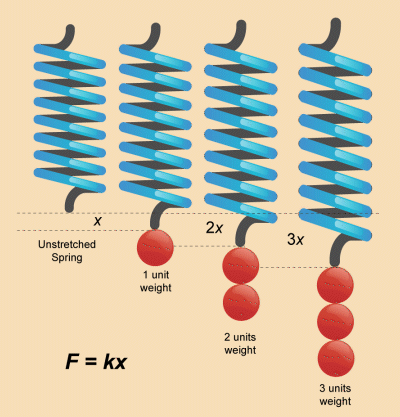
Here’s where Hooke’s Law and linear functions intersect. Adding another unit of that weight, for a total of 2 units of weight, stretches the spring by a comparable amount, 2x.
Then add a third weight, for a total of 3 units of weight, and the spring stretches by 3x amount. Then ask what will happen if you add 4 units of weight. Have students complete a pattern.
You can then take create a data table to show the linear pattern and common difference. Tie it all together with this graph, which shows the general graph of F = kx.
You can then connect the value of k with the slope of the line. Have students speculate what a high value of k corresponds to. With direct variations you should reinforce that there is a proportional relationship between the two variables. In the case of Hooke's Law, the force on the spring is proportional to the amount the spring stretches. In fact, k is the constant of proportionality.
For a self-paced student activity on linear functions that focuses on Hooke's Law, take a look at our Classroom Module, linked here: https://www.media4math.com/classroom/browse-modules/applications-linear-functions-hookes-law/preview.
Or take a look at our other resources on Hooke's Law: https://www.media4math.com/library/search?keys=Hooke%27s+Law&type=All