Display Title
Definition--Closure Property Topics--Rational Numbers and Closure: Multiplication
Display Title
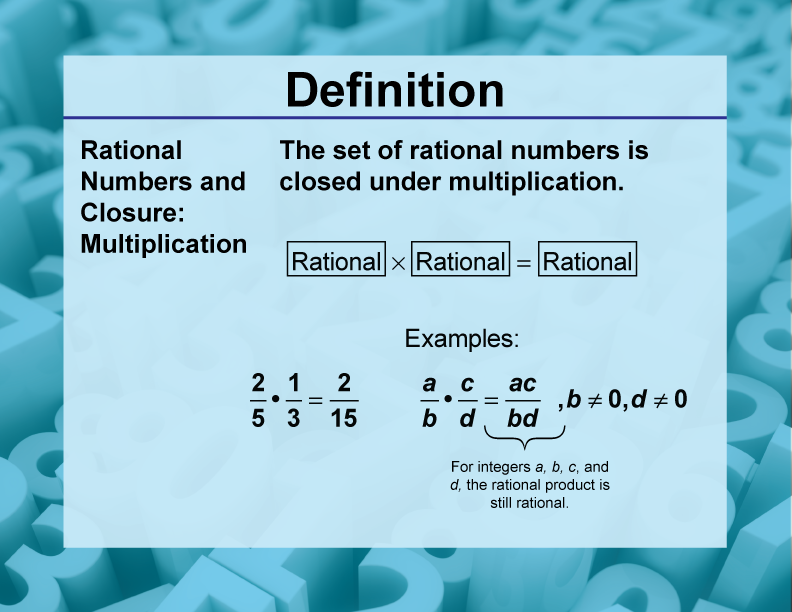
Definition--Closure Property Topics--Rational Numbers and Closure: Multiplication
Definition--Closure Property Topics--Rational Numbers and Closure: Multiplication
This is part of a collection of definitions on the topic of closure. This collection includes closure under the four basic operations, as well as for even numbers, odd numbers, whole numbers, integers, fractions, rational numbers, real number, and complex numbers.
Note: The download is a PNG file.
Related Resources
To see additional resources on this topic, click on the Related Resources tab.
Create a Slide Show
Subscribers can use Slide Show Creator to create a slide show from the complete collection of math definitions on this topic. To see the complete collection of definitions, click on this Link.
To learn more about Slide Show Creator, click on this Link.
Accessibility
This resources can also be used with a screen reader. Follow these steps.
-
Click on the Accessibility icon on the upper-right part of the screen.

-
From the menu, click on the Screen Reader button. Then close the Accessibility menu.

-
Click on the PREVIEW button on the left and then click on the definition card. The Screen Reader will read the definition.
| Common Core Standards | CCSS.MATH.CONTENT.HSN.RN.B.3, CCSS.MATH.CONTENT.HSN.CN.A.2 |
|---|---|
| Grade Range | 9 - 12 |
| Curriculum Nodes |
Algebra • The Language of Math • Numerical Expressions |
| Copyright Year | 2021 |
| Keywords | Closure Property |